In
the previous section we derived
the complete details about the rectangular portion. In this section
we will see the parabolic portion. The
parabolic portion has an area of 0.447fck
(8/21)xu (see derivation here).
This area multiplied by the width 'b' of the section will give the
volume of the parabolic stress block. And this volume is the compressive
force C2 contributed by the
parabolic portion. So we get
The distance of the centroid of this block (the point of application of C2) from the top most compression fibre = (9/14)xu
Taking moments of the forces C1 and C2 about the top most compression fibre, we get:
Eq.3.5
Where
Eq.3.6
Cu = C1 + C2 , the resultant compressive force, and,
x3 is the point of application of Cu from the top most compression fibre.
Substituting 3.3 and 3.4 in 3.6 we get
Cu = 0.447fck b (3/7)xu + 0.447fck b (8/21)xu
⇒ Cu = 0.447fck b (17/21)xu
⇒ Eq.3.7
Cu = 0.362 fck b xu
It may be noted that the above eq.3.7 is given in another form in the assumption c of cl.38.1 co the code. There, the area of the stress block is given as 0.362 fck xu . Multiplying this area by b, we get the volume, which is same as eq.3.7
Now we will derive an expression for x3:
Substituting 3.3, 3.4 and 3.7 in 3.5 we get:
0.447fckb(3/7)xu(3/14)xu+ 0.447fckb(8/21)xu(9/14)xu = 0.447fck b (8/21)xu x3
⇒ 0.447fck b (99/21x14)x2u = 0.447fck b (17/21)xu x3
⇒ (99/14)xu = 17 x3
⇒ Eq.3.8
x3 = (99/238)xu = 0.416xu
This is the same expression for 'depth of center of compressive force from the extreme fibre in compression' in assumption c of the code.
So we will get the lever arm z as:
z = d - 0.416xu
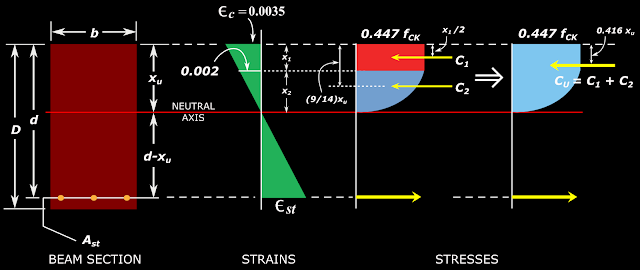
Thus we derived all the expressions required for the calculations of the quantities above the NA. Fig.3.12 below shows these details:
Fig.3.12
Complete details of stress block above NA
Eq.3.4
C2
= 0.447fck b (8/21)xu
The distance of the centroid of this block (the point of application of C2) from the top most compression fibre = (9/14)xu
Taking moments of the forces C1 and C2 about the top most compression fibre, we get:
Eq.3.5
Where
Eq.3.6
Cu = C1 + C2 , the resultant compressive force, and,
x3 is the point of application of Cu from the top most compression fibre.
Substituting 3.3 and 3.4 in 3.6 we get
Cu = 0.447fck b (3/7)xu + 0.447fck b (8/21)xu
⇒ Cu = 0.447fck b (17/21)xu
⇒ Eq.3.7
Cu = 0.362 fck b xu
It may be noted that the above eq.3.7 is given in another form in the assumption c of cl.38.1 co the code. There, the area of the stress block is given as 0.362 fck xu . Multiplying this area by b, we get the volume, which is same as eq.3.7
Now we will derive an expression for x3:
Substituting 3.3, 3.4 and 3.7 in 3.5 we get:
0.447fckb(3/7)xu(3/14)xu+ 0.447fckb(8/21)xu(9/14)xu = 0.447fck b (8/21)xu x3
⇒ 0.447fck b (99/21x14)x2u = 0.447fck b (17/21)xu x3
⇒ (99/14)xu = 17 x3
⇒ Eq.3.8
x3 = (99/238)xu = 0.416xu
This is the same expression for 'depth of center of compressive force from the extreme fibre in compression' in assumption c of the code.
So we will get the lever arm z as:
z = d - 0.416xu
Thus we derived all the expressions required for the calculations of the quantities above the NA. Fig.3.12 below shows these details:
Fig.3.12
Complete details of stress block above NA
Stress-Strain curve for steel
Note that the quantity xu (the depth of NA) is still an unknown. We have not derived an expression for it yet. We will now discuss about the steel. We want to know how the steel will be behaving at the point of impending failure. While we discuss about it, we will get the expression for xu also.
We
want the stress in steel. We must calculate it using the graph in
fig.23 of the code. So let us first discuss about fig.23. Fig.23A
gives the stress strain curve for cold worked bars like Fe415 and
Fe500. And Fig.23B gives the stress strain curve for mild steel like
Fe250.
These graphs are plotted by conducting tension tests on steel. Strains are plotted along the X-axis, and stresses are plotted along the Y-axis. For mild steel, there is a definite yield point. That is., there is a particular stress after which the steel will begin to yield. So after this point the steel will continue to elongate at constant stress. Thus the graph after this point will be horizontal. The stress at the yield point is taken as the characteristic strength fy of steel. We have to apply the partial safety factor to this characteristic value to get the 'design value'. So we divide fy by 1.15 giving fy/1.15 = 0.87fy. The lower curve is obtained by dividing all the values by 1.15, and so, it is the design curve. If we know the strain in steel, we can calculate the design value of stress in that steel by using the design curve.
As we did in the case of concrete, we can now note down the effect of reducing the stress in steel from fy to 0.87fy: In an analysis problem, the steel is considered to have yielded if the stress in it is 0.87fy. If it was fy instead of 0.87fy, we can expect the steel to take more load before it yields.
In a design problem, we can apply only that load which cause a stress of 0.87fy. If it was fy instead of 0.87fy, we can apply a greater load. But that is not the case. We must consider only 0.87fy.
For cold worked bars, there is no definite yield point. That is., there is no particular stress after which the steel will elongate at constant stress. So the change from the inclined graph to the horizontal graph do not take place at a particular point. Instead, the change takes place gradually through some distance. Because of this, it is not easy to point out a particular value as the characteristic strength. So we follow the procedure given by the code to obtain the characteristic strength from the graph.
The code specifies that the 'stress corresponding to 0.002 strain offset' should be taken as the characteristic strength fy. This can be explained as follows: Consider a tensile test being conducted on a steel specimen. When the applied stress increases, the strain also increases. We plot the strain along the X axis and the stress along the Y axis. Initially this graph is a straight line. The slope of this line = Stress/Strain = Es, the modulus of elasticity of steel. But as the stress increases, it will begin to take a curved shape. With further increase of stress, the graph will become horizontal. After becoming horizontal, it will continue to elongate at constant stress. So when we reach the horizontal portion, we can be sure that the yielding have begun. But, because of the curved region between the inclined and horizontal graphs, we cannot find a particular value of stress at which the yielding began.
To solve this, we make use of a special property of steel: The property of 'giving a residual strain after yielding'. That is., while doing the tension test, if we release the load while in the initial inclined region, the steel will contract back to it's original length. This is because, the initial inclined region is the 'elastic' region. But after yielding, if the load is released, the steel will not attain it's original length. There will be a residual strain. So it follows that, if we release the load at a particular value of stress, and if on release, a residual strain is obtained, we can be sure that the steel has yielded. But still, we do not know the particular value of stress upon the release of which, the steel will begin to give residual strains. Also, the residual strain can be any value. It can be 0.001, 0.002, 0.0025, or any similar value. Whatever be the value, if there is a residual strain, we can be sure that yielding has begun. In order that all of us use a same standard value, the code specifies the required value of residual strain as 0.002. So we want the 'value of the stress', upon the release of which, the steel will give a residual strain of 0.002.
This can be easily determined from the stress strain curve of steel. We continue the test as usual. There is no need for unloading at any point. After plotting the graph, we draw a line parallel to the initial inclined line. And this parallel line should pass through the 0.002 point on the X-axis. The point of intersection of this line with the orginal graph gives the 'characteristic yield strength' fy of steel. As usual, we divide all the stress values by the partial safety factor 1.15, to get the design curve. The point of intersection of the parallel line with the design curve will give the 'design yield strength' of steel.
For analysis and design purposes, we can use the graph in fig.23A of the code. We will try to plot a design curve on our own. While trying to plot it, we will understand it's salient features. Let us plot the design curve for Fe415 steel.
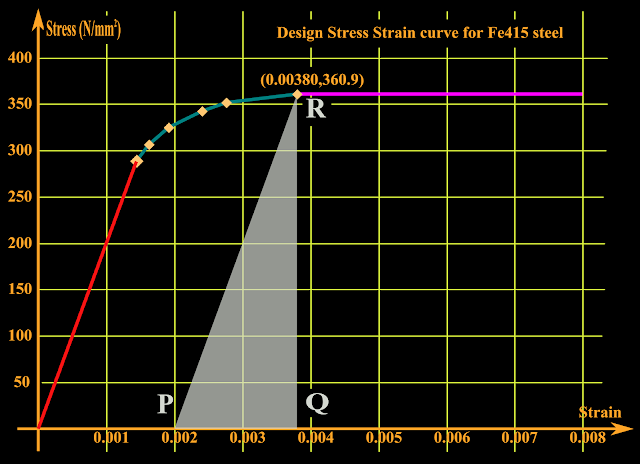
We know that the initial inclined portion and the final horizontal portions are straight lines. So they can be easily plotted. For the inclined line, any two points on it is sufficient, and for the horizontal portion, any one point on it is sufficient. The curved portion is the difficult part. It requires more points. The fig.23A gives us these points. We can see a dashed line parallel to the initial inclined line, starting from 0.002 on the X axis. It meets the characteristic curve at a certain point. A horizontal dashed line is drawn from this meeting point towards the left up to the Y axis. At the point of intersection of the horizontal dashed line and the Y axis is marked: 'fy'. This means that the 'y coordinate of the point of intersection' of the inclined dashed line with the characteristic curve is fy. (It will indeed be fy, the characteristic yield strength, because 0.002 is the 'proof strain' as discussed earlier). So the y coordinate of the corresponding point on the design curve will be equal to fy/1.15 = 415/1.15 = 360.9 N/mm2. Now we want the x coordinate. For this we will use the fig.3.13 given below:
Fig.3.13
Last point of the curved portion
In the fig., the initial inclined line is drawn in red colour, the final horizontal line is drawn in magenta colour, and the intermdiate curve is drawn in blue colour. A triangle PQR is drawn. PR corresponds to the inclined dashed line in the code, drawn from the strain value of 0.002. So we can see that the x coordinate will be equal to 0.002 + PQ. Thus our next step is to calculate PQ.
In the ΔPQR, tan∠RPQ = RQ⁄PQ
But tan∠RPQ is the slope of PR. So it is also the slope of the initial inclined line of the design curve.
These graphs are plotted by conducting tension tests on steel. Strains are plotted along the X-axis, and stresses are plotted along the Y-axis. For mild steel, there is a definite yield point. That is., there is a particular stress after which the steel will begin to yield. So after this point the steel will continue to elongate at constant stress. Thus the graph after this point will be horizontal. The stress at the yield point is taken as the characteristic strength fy of steel. We have to apply the partial safety factor to this characteristic value to get the 'design value'. So we divide fy by 1.15 giving fy/1.15 = 0.87fy. The lower curve is obtained by dividing all the values by 1.15, and so, it is the design curve. If we know the strain in steel, we can calculate the design value of stress in that steel by using the design curve.
As we did in the case of concrete, we can now note down the effect of reducing the stress in steel from fy to 0.87fy: In an analysis problem, the steel is considered to have yielded if the stress in it is 0.87fy. If it was fy instead of 0.87fy, we can expect the steel to take more load before it yields.
In a design problem, we can apply only that load which cause a stress of 0.87fy. If it was fy instead of 0.87fy, we can apply a greater load. But that is not the case. We must consider only 0.87fy.
For cold worked bars, there is no definite yield point. That is., there is no particular stress after which the steel will elongate at constant stress. So the change from the inclined graph to the horizontal graph do not take place at a particular point. Instead, the change takes place gradually through some distance. Because of this, it is not easy to point out a particular value as the characteristic strength. So we follow the procedure given by the code to obtain the characteristic strength from the graph.
The code specifies that the 'stress corresponding to 0.002 strain offset' should be taken as the characteristic strength fy. This can be explained as follows: Consider a tensile test being conducted on a steel specimen. When the applied stress increases, the strain also increases. We plot the strain along the X axis and the stress along the Y axis. Initially this graph is a straight line. The slope of this line = Stress/Strain = Es, the modulus of elasticity of steel. But as the stress increases, it will begin to take a curved shape. With further increase of stress, the graph will become horizontal. After becoming horizontal, it will continue to elongate at constant stress. So when we reach the horizontal portion, we can be sure that the yielding have begun. But, because of the curved region between the inclined and horizontal graphs, we cannot find a particular value of stress at which the yielding began.
To solve this, we make use of a special property of steel: The property of 'giving a residual strain after yielding'. That is., while doing the tension test, if we release the load while in the initial inclined region, the steel will contract back to it's original length. This is because, the initial inclined region is the 'elastic' region. But after yielding, if the load is released, the steel will not attain it's original length. There will be a residual strain. So it follows that, if we release the load at a particular value of stress, and if on release, a residual strain is obtained, we can be sure that the steel has yielded. But still, we do not know the particular value of stress upon the release of which, the steel will begin to give residual strains. Also, the residual strain can be any value. It can be 0.001, 0.002, 0.0025, or any similar value. Whatever be the value, if there is a residual strain, we can be sure that yielding has begun. In order that all of us use a same standard value, the code specifies the required value of residual strain as 0.002. So we want the 'value of the stress', upon the release of which, the steel will give a residual strain of 0.002.
This can be easily determined from the stress strain curve of steel. We continue the test as usual. There is no need for unloading at any point. After plotting the graph, we draw a line parallel to the initial inclined line. And this parallel line should pass through the 0.002 point on the X-axis. The point of intersection of this line with the orginal graph gives the 'characteristic yield strength' fy of steel. As usual, we divide all the stress values by the partial safety factor 1.15, to get the design curve. The point of intersection of the parallel line with the design curve will give the 'design yield strength' of steel.
For analysis and design purposes, we can use the graph in fig.23A of the code. We will try to plot a design curve on our own. While trying to plot it, we will understand it's salient features. Let us plot the design curve for Fe415 steel.
We know that the initial inclined portion and the final horizontal portions are straight lines. So they can be easily plotted. For the inclined line, any two points on it is sufficient, and for the horizontal portion, any one point on it is sufficient. The curved portion is the difficult part. It requires more points. The fig.23A gives us these points. We can see a dashed line parallel to the initial inclined line, starting from 0.002 on the X axis. It meets the characteristic curve at a certain point. A horizontal dashed line is drawn from this meeting point towards the left up to the Y axis. At the point of intersection of the horizontal dashed line and the Y axis is marked: 'fy'. This means that the 'y coordinate of the point of intersection' of the inclined dashed line with the characteristic curve is fy. (It will indeed be fy, the characteristic yield strength, because 0.002 is the 'proof strain' as discussed earlier). So the y coordinate of the corresponding point on the design curve will be equal to fy/1.15 = 415/1.15 = 360.9 N/mm2. Now we want the x coordinate. For this we will use the fig.3.13 given below:
Fig.3.13
Last point of the curved portion
In the fig., the initial inclined line is drawn in red colour, the final horizontal line is drawn in magenta colour, and the intermdiate curve is drawn in blue colour. A triangle PQR is drawn. PR corresponds to the inclined dashed line in the code, drawn from the strain value of 0.002. So we can see that the x coordinate will be equal to 0.002 + PQ. Thus our next step is to calculate PQ.
In the ΔPQR, tan∠RPQ = RQ⁄PQ
⇒ PQ = RQ⁄tan∠RPQ
But tan∠RPQ is the slope of PR. So it is also the slope of the initial inclined line of the design curve.
But the slope of this initial
inclined line =
Stress⁄Strain = Elastic modulus of steel = Es = 2 x
105 N/mm2.
We know that RQ = the y coordinate of the point R, which we already calculated as 360.9
Thus we get PQ = 360.9⁄200000 = 0.0018045
So the x coordinate of point R = 0.002 + 0.0018045 = 0.0038045 = 0.00380
Thus the coordinates of the last point of the curved portion are (0.00380,360.9)
This is for Fe415 steel. For the general case, for the last point of the curved portion, we can write the following:
Eq.3.9
x coordinate = 0.002 + [fy/ 1.15 x 200000] = 0.002 + fy/230000
y coordinate = fy/ 1.15
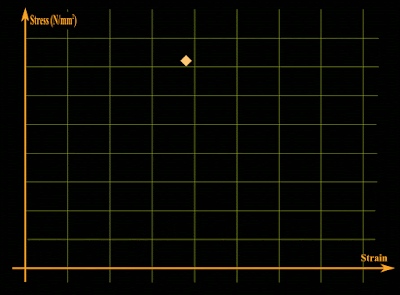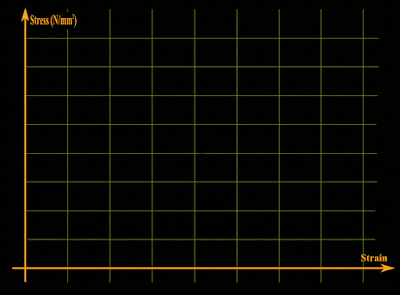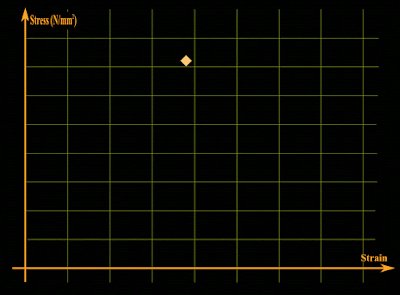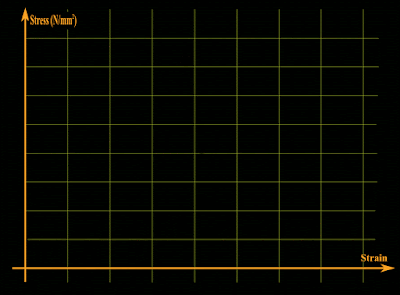
The above discussion was based on fig.3.13 above. It is a complete fig. with all the required points of the design curve of Fe415 steel. Such a complete fig. is used for discussion purpose only. Based on that discussion, we have derived the general form in Eq.3.9. So, on a new empty graph paper, with only the axes drawn to proper scale, we can directly mark the last point of the curved portion using Eq.3.9. This is shown in the animation below:
This is also the first point of the final horizontal portion. As the portion is horizontal, it requires only one point to plot. So with this just one point, the calculations related to the final horizontal line is complete.
Now we will calculate the second last point of the curved portion. We will do this in the next section.
Thus we get PQ = 360.9⁄200000 = 0.0018045
So the x coordinate of point R = 0.002 + 0.0018045 = 0.0038045 = 0.00380
Thus the coordinates of the last point of the curved portion are (0.00380,360.9)
This is for Fe415 steel. For the general case, for the last point of the curved portion, we can write the following:
Eq.3.9
x coordinate = 0.002 + [fy/ 1.15 x 200000] = 0.002 + fy/230000
y coordinate = fy/ 1.15
The above discussion was based on fig.3.13 above. It is a complete fig. with all the required points of the design curve of Fe415 steel. Such a complete fig. is used for discussion purpose only. Based on that discussion, we have derived the general form in Eq.3.9. So, on a new empty graph paper, with only the axes drawn to proper scale, we can directly mark the last point of the curved portion using Eq.3.9. This is shown in the animation below:
This is also the first point of the final horizontal portion. As the portion is horizontal, it requires only one point to plot. So with this just one point, the calculations related to the final horizontal line is complete.
Now we will calculate the second last point of the curved portion. We will do this in the next section.
Copyright ©2015 limitstatelessons.blogspot.com- All Rights Reserved
No comments:
Post a Comment